Fraunhofer Institute for Industrial Mathematics ITWM
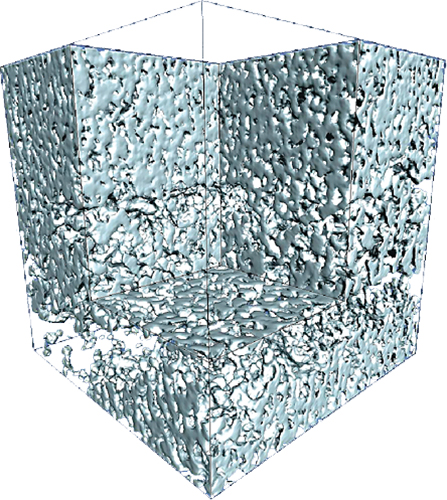
Firn is sintered snow and forms the top layer of polar ice sheets. Its micro structure reflects the climate of the time when the layer formed. Firn core B26 was drilled during the North Greenland traverse of the Alfred-Wegener-Institut für Polar- und Meeresforschung Bremerhaven in1995. Five samples from different depths within the core were imaged using CT. For the segmented pore system the structure model index (SMI) was computed - a shape factor for structure components derived from volume fraction, specific surface area and density of the integral of mean curvature. With increasing depth, the SMI indicates a transition from cylindrical towards isolated spherical pores.
At around 50-100 m depth below surface, the formerly interconnected air-filled pore space between ice grains becomes isolated and creates spherical bubbles. The statistical analysis of the 3D point pattern of bubble center locations is proven (Redenbach, 2009) to give information about the thinning of the ice over depth, which is an important input for the dating of the ice which is in turn important to give a correct interpretation to the ‘climate archive’ stored in the ice cores.
Publications
- Kronenberger, M.; Wirjadi, O.; Freitag, J.; Hagen, H.:
Gaussian Curvature Using Fundamental Forms for Binary Voxel Data.
Graphical Models, 82: 123-136, 2015.
https://doi.org/10.1016/j.gmod.2015.06.009 - J. Ohser, C. Redenbach, K. Schladitz:
Mesh Free Estimation of the Structure Model Index.
Image Analysis & Stereology 28, S. 101-107, 2009.
- C. Redenbach, A. Särkkä, J. Freitag, K. Schladitz:
Anisotropy analysis of pressed point processes.
Advances in Statistical Analysis 93(3), S. 237ff, 2009.
- C. Redenbach, A. Särkkä, M. Sormani:
Classification of Points in Superpositions of Strauss and Poisson Processes,
Spatial Statistics 12, Pages 81-95, 2015.
- T. Rajala, A. Särkkä, C. Redenbach, M. Sormani:
Estimating Geometric Anisotropy in Spatial Point Patterns,
Spatial Statistic 15, Pages 100-114, 2016.